Observations relating to an insulation joint test carried out in the field
Reported insulating flange resistance = 2,9 Mega Ohms
If this was a straight measurement using a multi-meter this will include the passage of the measuring current through earth.
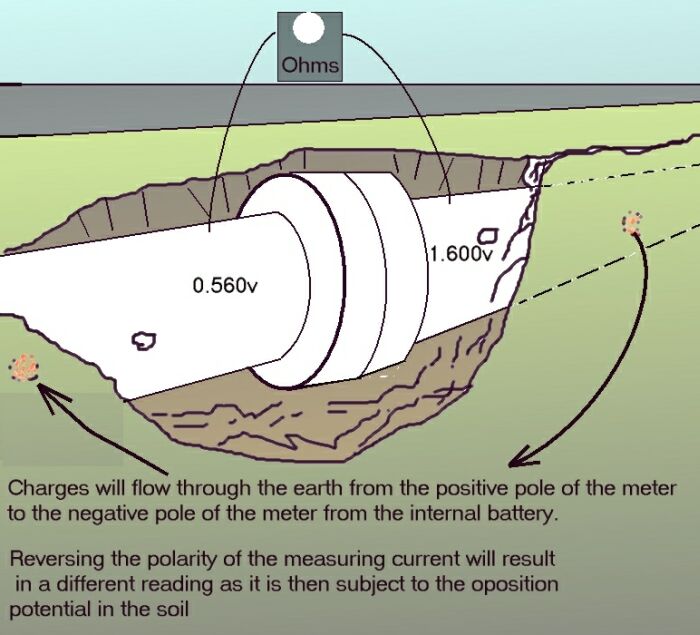
The first picture shows the potentials of the pipe metal on one side of the isolation joint being higher than the potential of the pipe metal on the other side. This means that the charges will tend to equalise through the joint, and equilibrium will be reached with current flowing. If there is total resistance in the joint and the coating is perfect then equilibrium will be reached without current flowing through the system.
In practical terms this means that the pipe metal with the higher potential will send charges to the lower potential through the ground if the isolation joint is an absolute resistance. Where it enters the electrolyte it will disolve metal in proportion to the current. If the 'unprotected' side of the joint is a manifold or pipework on a concrete base the charges will still seek to equalise through any available path according to Kirchoff's laws.
We cannot measure the resistance of the earth path as this includes the increasing number of resistances in parallel on the way out of the metal and the decreasing number of resistances in parallel on the way back in.
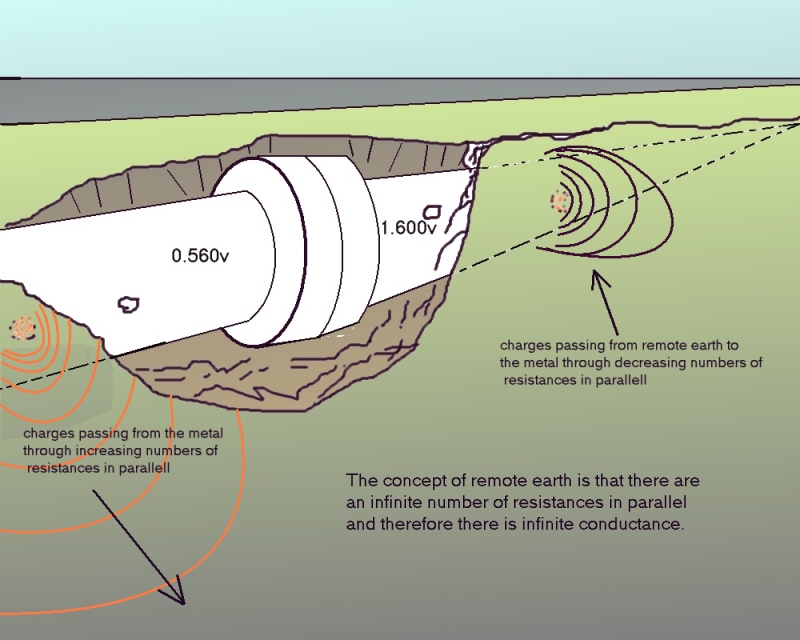
The driving force in the meter is 9 volts but cannot apply Ohms law in the usual way to determine the resistance in the measuring circuit. we can never know the exact surface area or location of the pipe metal to earth interface/s.
The surface area of the coating fault determins the amount of current caused by the corrosion reaction at the active interface and the resistance at the other. The reading on the meter is the result of the sum of all componentsin the measuring circuit so we can not use the 'amps' value in Ohms law to calculate the earth resistance. All we can measure is the total measuring circuit resistance and that includes an EMF that is directional and therefore polarity must be considered an any calculation.
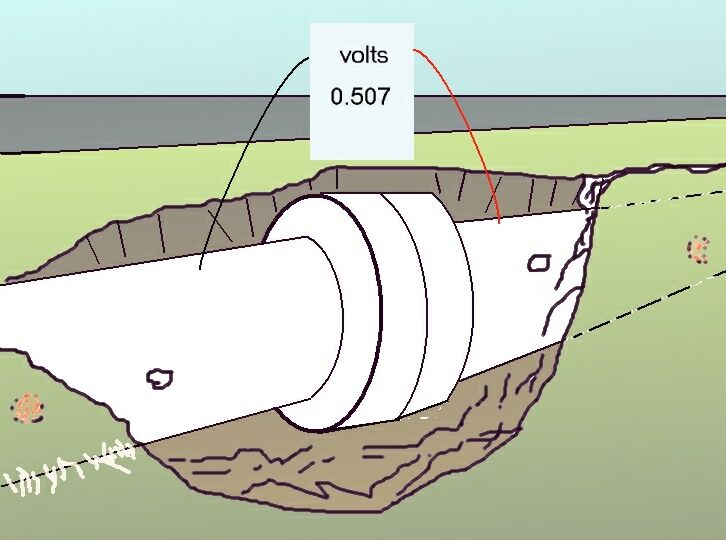
Dc Voltage across Flange 0.507 Volts
The DC voltage across the flange shows the state of equilibrium, but this can include the volts drop through the 2.9 mega-ohms of the flange itself, if there is a path through a broken insulation washer or sleeve. The amount of current leakage would be very small to render 0.507 volts (IR drop).
Pipe to soil on CP side of flange -1.5 Volts
Pipe to soil on opposite side -0.795 Volts
If these two were measured with reference to a single electrode in a fixed position then the voltage across the flange must be the difference between the two. (ie 0.705 volts.)
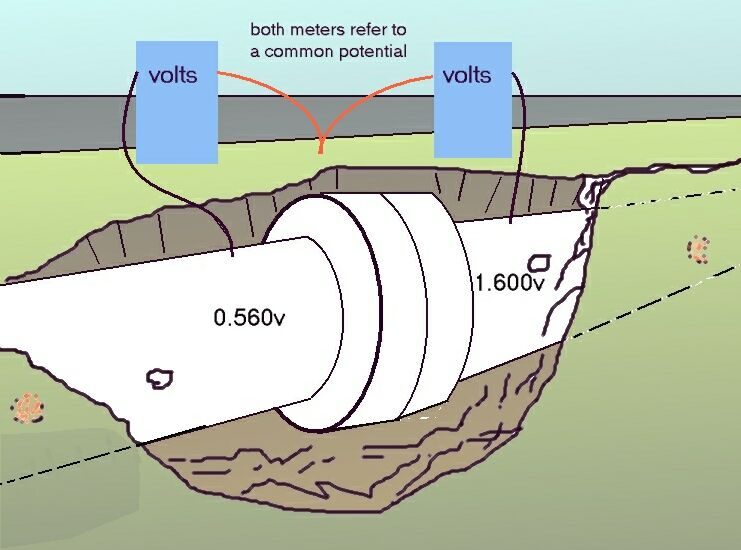
Let us assume that the electrode was not moved then there is a potential difference to be accounted for of either
0.507 + 0.795 = 1.302 volts or
0.795 -0.507 = 0.288 volts
These readings can easily be demonstrated on the models in the demonstration room using a multimeter on Ohms setting.
If the half-cell is moved then we have two irreconcilable voltages
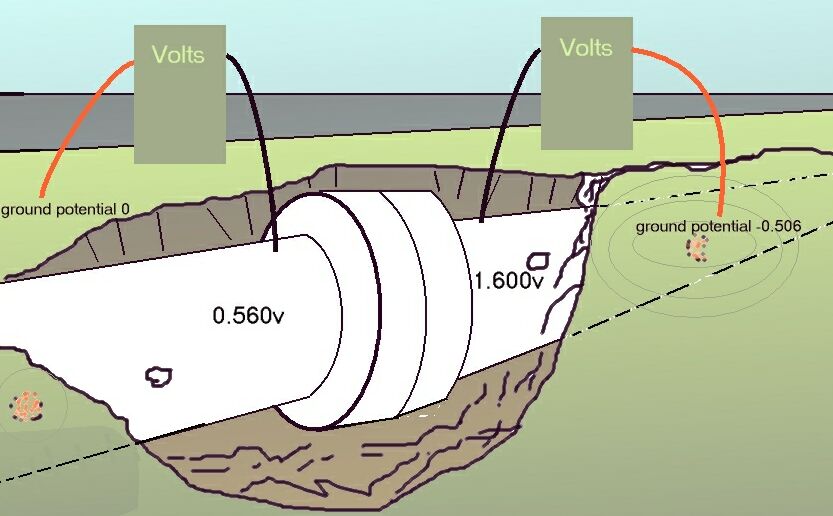
Consequently, the only way to measure the actual resistance across an insolation flange is by measuring bolt by bolt to each side if the flange.
There is no way of measuring the resistance of a manufactured insulating joint once it has been welded to the main pipeline.





